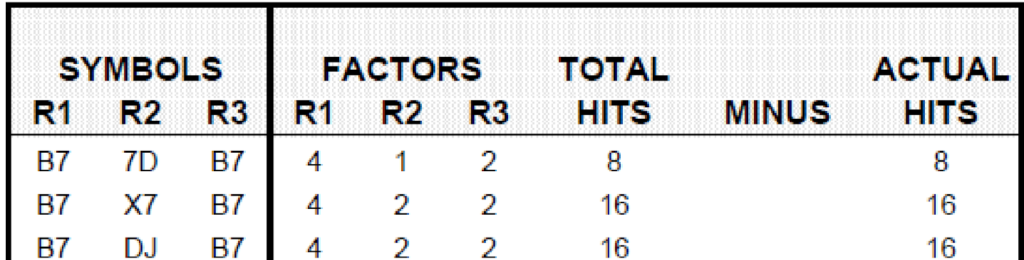
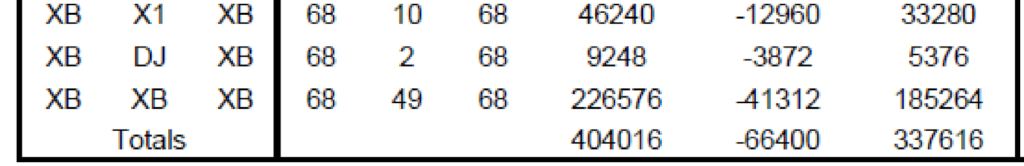
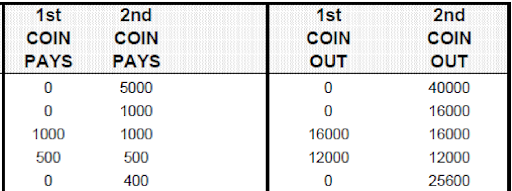
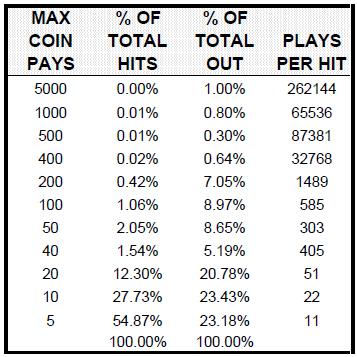
About the Author
Author: Dr Catalin Barboianu PhD
Catalin Barboianu PhD is a games mathematician and problem-gambling researcher. He authored ten books on gambling mathematics and several academic articles in the field of problem gambling and philosophy of science. Catalin is a science writer and consultant for the mathematical aspects of gambling for the gaming industry and problem-gambling institutions. Read More